Standard methods for measuring the permeability of oxygen and carbon dioxide gas and water vapor through flat polymer structures are described in ASTM D 3985-81 and E 96-80, respectively. The measurement of permeability of organic vapors has been the subject of numerous investigations. Commercial equipment for measuring the permeability of organic vapors through plastic films are now available from MAS Technologies Inc. (Zumbrota, MN) and Modern Controls Inc. (Minneapolis, MN).
Methods for determining permeability are of two types: continuous flow and lag-time or quasi-isostatic.
Continuous-flow Method. Figure 1 shows a schematic of a continuous-flow-method setup for measuring oxygen permeability in the presence of water vapor.
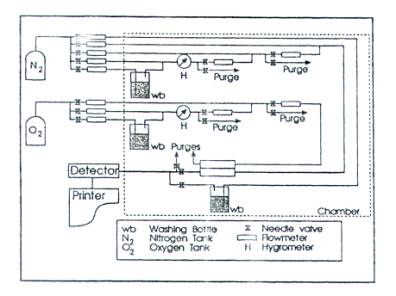
FIGURE 1 Continuous flow permeation method. (Reprinted with permission from R. Gavara and R. J. Hernandez. J. Polym. Sci., Part B, 32, 2375, 1994.)
The solution to Fick's second law from a continuous-flow permeation experiment with D independent of concentration is given by
 (1)
(1)
where Ft is the flow rate of the permeant through the film sample and Fss is the steady-state flow. A typical curve obtained using the continuous-flow method is presented in Fig. 2.
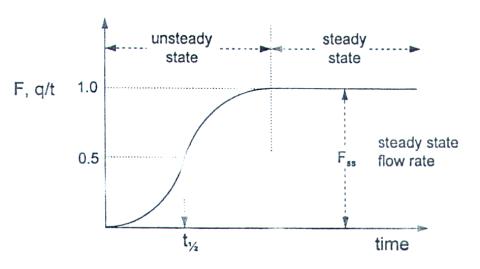
FIGURE 2 Flow profile in a continuous-flow method.
The experimental permeation flow up to a value of the flow ratio of 0.95 can be virtually described by the first term of Eq.1:
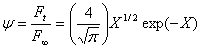 (2)
(2)
where X=l/4Dt. The Newton-Rhapson method can be used to evaluate X versus t in Eq.2, and the diffusion coefficient D is obtained from the slope of the linear fitting of X-1 versus t for 0.05< ψ <0.95. The permeability coefficient P is calculated from the value of F ss ,
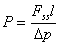 (3)
(3)
where Δp is the partial pressure across the film during the permeation experiment. Simple constant diffusion coefficient D can be approximately calculated by
 (4)
(4)
where t1/2 is the time required at Ft /Fss =0.5.
Gavara and Hernandez have presented a simple method to analyze the consistency of experimental Fickian permeability data.
Lag-Time Method. Figure 3 shows an apparatus for measuring the permeation and sorption of oxygen in polymers films.
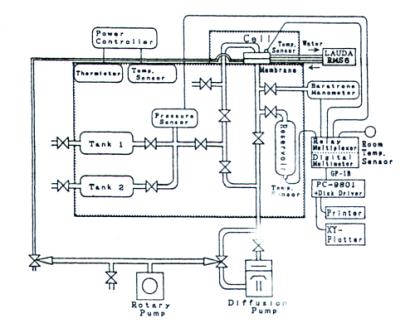
FIGURE 3 Lag-time permeation apparatus. (Reprinted with permission from K. Toi, H. Suzuki, I. Ikenasto, T. Ito, and
T. Kasi, J. Polym. Sci., Part B, 33, 777, 1995.)
The solution of Fick's second law when the initial concentration of permeant in the film is zero is
 (5)
(5)
where Q(l) is the quantity of permeant that has passed at x=l per unit of the membrane area during time t. A plot of Eq.5 is presented in Fig. 4, where the partial pressure is plotted versus time t. At the steady state, at very large t values, Q is given by
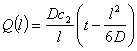 (6)
(6)
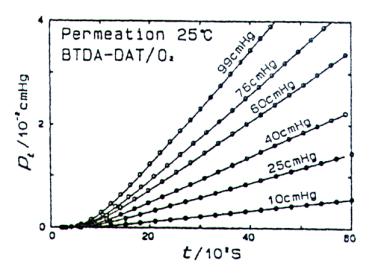
FIGURE 4 Plot of lag-time permeation experiment. (Reprinted with permission from K. Toi, H. Suzuki, I. Ikenasto, T. Ito, and T. Kasi, J. Polym. Sci., Part B, 33, 777, 1995.)
The intercept of the steady-state line with the t axis, which occurs at Q(l)=0, defines the lag time θ :
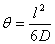 (7)
(7)
A quasi-isostatic (lag-time) method has been used by Wahid for measuring organic vapor permeability through metallocene polymer films.
For a penetrant/polymer system that follows the dual-mode sorption model of Eqs. 8 and 9,
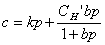 (8)
(8)
 (9)
(9)
the lag-time is given by the following expression:
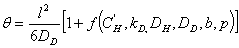 (10)
(10)
where f is a complex function.
